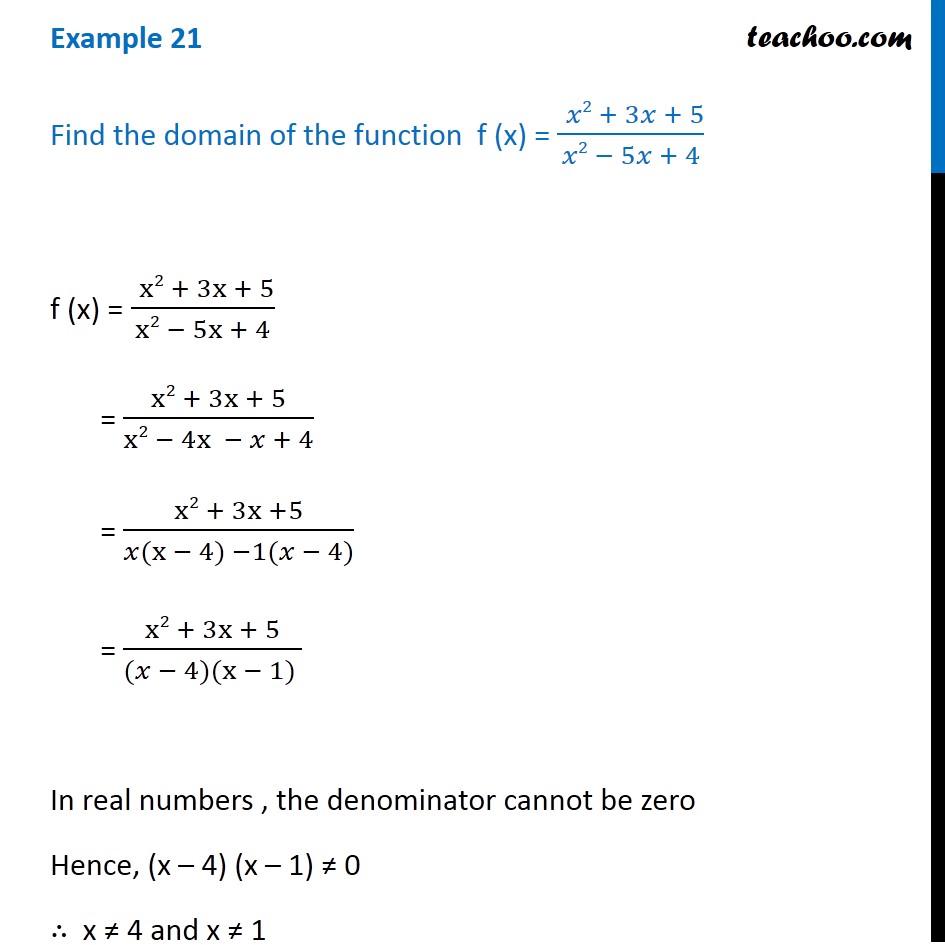
SOLUTION find the range and domain f (x)=1/x2 Question find the range and domain You can put this solution on YOUR website!Algebra Find the Domain and Range f (x)=3^x f (x) = 3x f ( x) = 3 x The domain of the expression is all real numbers except where the expression is undefined In this case, there is no real number that makes the expression undefined Interval NotationDetermine the equation of the vertical asymptote for the graph of this function, and state the domain and range of this functionf(x) = ln(x 1) 3 asked in Mathematics by Reckless A Vertical asymptote x = 3;

3 6 Warm Up Find The Initial Point State The Domain Range And Compare To The Parent Function F X X Y 3 X 1 Y 1 2 X Y X 1 Ppt Video Online Download
The range and domain of a function f(x)=3/x+1
The range and domain of a function f(x)=3/x+1-Answer and Explanation 1 Become a Studycom member to unlock this answer!Functions assign outputs to inputs The domain of a function is the set of all possible inputs for the function For example, the domain of f (x)=x² is all real numbers, and the domain of g (x)=1/x is all real numbers except for x=0 We can also define special functions whose domains are more limited Google Classroom Facebook Twitter




3 6 Warm Up Find The Initial Point State The Domain Range And Compare To The Parent Function F X X Y 3 X 1 Y 1 2 X Y X 1 Ppt Video Online Download
The domain and range of f −1 f −1 are given by the range and domain of f, f, respectively Therefore, the domain of f −1 f −1 is 0, ∞) 0, ∞) and the range of f −1 f −1 is −1, ∞) −1, ∞) To find a formula for f −1, f −1, solve the equation y = (x 1) 2 y = (x 1) 2 for x x If y = (x 1) 2, y = (x 1 For instance, f (x) = The domain is simply the denominator set equal to 0, {xl x≠3} However, range is found by solving for (isolating x to one side) and setting the denominator equal to zero x = So range is {xl x≠0} This is a systematic method that I assume is the only way to find the range Assuming you meant f (x)=2x*4^ (x1)3 the domain of all polynomials (2x) is (∞,∞) the domain of all exponentials is (∞,∞) so, the domain of f (x) is likewise (∞,∞) now work with the ranges in like wise x > (xh) translates right by h y > (yk) translates up by k
Mathy=1\mid x3 \mid\tag*{}/math math\implies \mid x3 \mid \geq 0\tag*{}/math math\implies \mid x3 \mid \leq 0\tag*{}/math math\implies 1\mid x3 Denominator (3x)=0 x=3 This means at x=3 function is not defined And by definition of domain The domain is where the function is not defined Domain is Range Put f(x)=y Range is the set of value that correspond to domain Equate the denominator to zero Denominator (y1)=0 y=1 This means at y=1 function is not defined Range isF(x)=x3 _____ x^25x66 f(x) = Factor the denominator f(x) = The domain contains all numbers which can be substituted for x, and an answer is defined Denominators cannot be 0 so x cannot be any number that will cause the denominator to be 0
How To Given a function written in equation form, find the domain Identify the input values Identify any restrictions on the input and exclude those values from the domain Write the domain in interval form, if possible Example 332 Finding the Domain of a Function Find the domain of the function f(x) = x2 − 1Y=f(x) The x is to be multiplied by 1 This makes the translation to be "reflect about the yaxis" while leaving the ycoordinates alone y=1/2 f(x/3) The translation here would be to "multiply every ycoordinate by 1/2 and multiply every xcoordinate by 3" y=2f(x)5 There could beThe graph of the function f(x)=(x3)(x1) is shown belowWhich is true about the domain and range of the function Mathematics Asked 1 day ago Give Answer Answers we have Convert into vertex formGroup terms that contain the same variable, and move the constant to the opposite side of the equation Complete the square
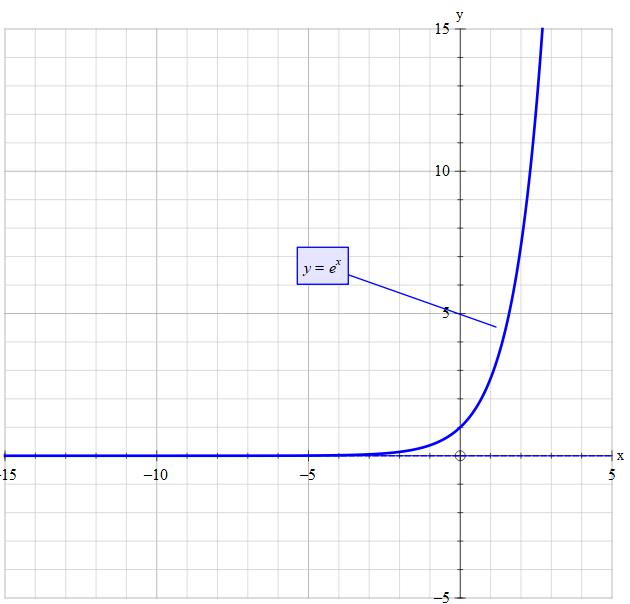



What Is The Domain And Range Of Y E X Socratic
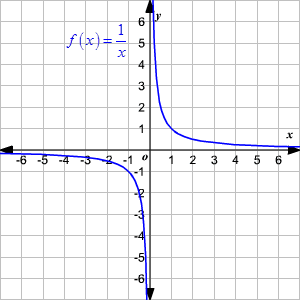



Domain And Range Of Rational Functions
–1 x 2 4 y 021 Mathematics Learning Centre, University of Sydney 5 State its domain and range Solution The function is defined for all real xThe vertex of the function is at (1,1) and therfore the range of the function is all real y ≥ 1 12 Specifying or restricting the domain of a functionFor the function f(x) = 2(x 3)^2 1, identify the vertex, domain, and range A) The vertex is (3, 1), the domain is all real numbers, and the range isExample 5 Find the domain and range of the following function f (x) = 2/ (x 1) Solution Set the denominator equal to zero and solve for x x 1 = 0 = 1 Since the function is undefined when x = 1, the domain is all real numbers except 1 Similarly, the range is all real numbers except 0
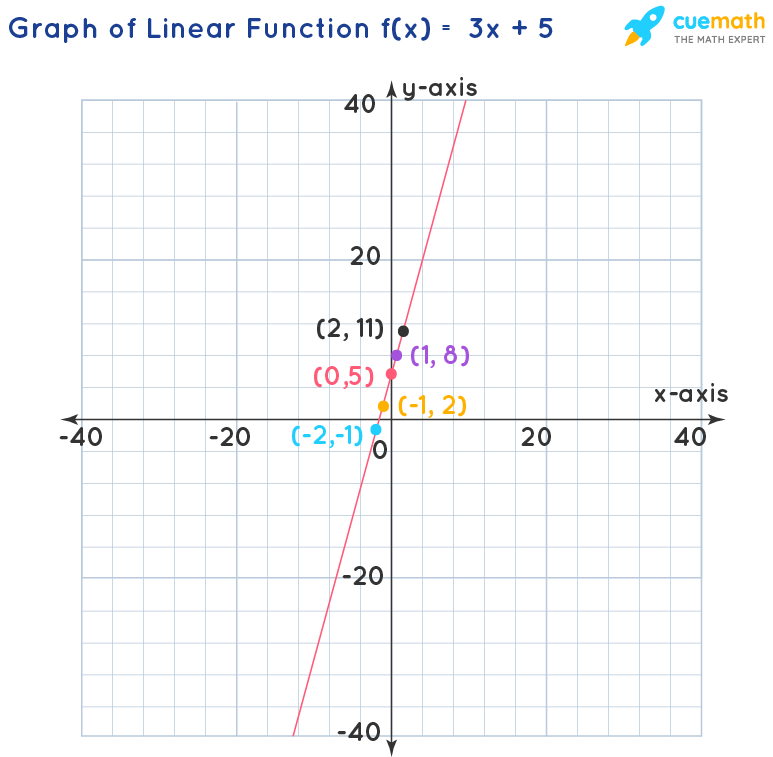



What Are The Domain And Range Of The Function F X 3 X 5 Solved



1 1 Webassign Answers
This is a quadratic function There are no rational or radical expressions, so there is nothing that will restrict the domain Any real number can be used for x to get a meaningful output Because the coefficient of x 2 is negative, it will open downward Ra the funtion f (x) =3 (x1)^2 2 identify the vertex, domain and range Categories MathematicsExplain 1 Identifying a Function's Domain, Range and End Behavior from its Graph Recall that the domain of a function fis the set of input values x, and the range is the set of output values f(x) The end behavior of a function describes what happens to the f(x)values as the xvalues either increase without bound



Solution F X 1 3 X What Would The Domain And Range Of This Function Be I Am Guessing That There Is One Asymptote Y 1 3 So Only The Range Has A Restriction
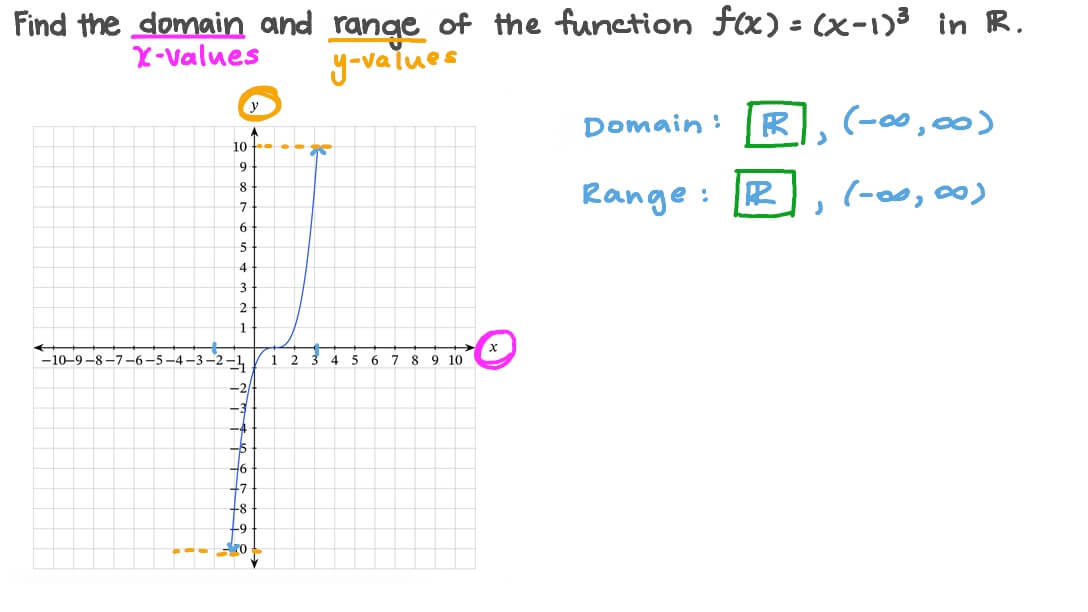



Question Video Finding The Domain And Range Of A Cubic Function Given Its Graph Nagwa
Domain and Range The domain of a function f ( x ) is the set of all values for which the function is defined, and the range of the function is the set of all values that f takes (In grammar school, you probably called the domain the replacement set and the range the solution set They may also have been called the input and output of the function) Find domain and range of f (x)= square root of (x 1) (3 x) Enter your answer for the domain using interval notation Set the radicand in greater than or equal to to find where the expression is defined Fx x 2 2 x 1 Solution to Example 3 Let fx4 on the square root of x 1 Identify the domain of the function fx x 3 Let f R– {(1/3)} → R be a function defined as f(x) = 4x/(3x 4) Show that, in f R– {(4/3)} → Range of f, f is oneone and onto




Domain And Range Free Math Help




What Is The Domain And Range Of F X Abs X 3 5 2 Socratic
Set the denominator equal to zero Remember, dividing by 0 is undefined So if we find values of x that make the denominator zero, then we must exclude them from the domain Now to find the range, notice that The graph of the function f(x) = –(x 3)(x – 1) is shown below What is true about the domain and range of the function?Find domains and ranges of the toolkit functions We will now return to our set of toolkit functions to determine the domain and range of each 11 Figure 11 For the constant function f ( x) = c \displaystyle f\left (x\right)=c f (x) = c, the domain consists of all real numbers;



Determine Domain And Range From A Graph College Algebra




The Graph Of The Function F X X 3 X 1 Is Shown Below What Is True About The Domain And Range Of Brainly Com
Domain and range The domain and range of a function is all the possible values of the independent variable, x, for which y is defined The range of a function is all the possible values of the dependent variable y The example below shows two different ways that a function can be represented as a function table, and as a set of coordinatesClick here👆to get an answer to your question ️ Find the domain and the range of the real function f defined by f (x) = √( ( x 1 ))Find the domain of function f given by f(x) = √(x 1) / (x 3) To find the domain of the above function we need two conditions condition (1) x 1 is under the square root and must be positive or zero Hencex 1 ≥ 0 leads to x ≤ 1 condition (2) x 3 is in the denominator and must be non zero Hence x must not take the value 3




Find The Domain And Range Of The Function F X 2 X 5



Find The Domain And Range Of The Function F X 1 X 5 Donimain
Domain and range » Tips for entering queries Enter your queries using plain English To avoid ambiguous queries, make sure to use parentheses where necessary Here are some examples illustrating how to ask for the domain and range domain of log(x) (x^21)/(x^21) domain;F (x) = x3 f ( x) = x 3 The domain of the expression is all real numbers except where the expression is undefined In this case, there is no real number that makes the expression undefined Interval Notation (−∞,∞) ( ∞, ∞) Set Builder Notation {xx ∈ R} { x x ∈ ℝ } The rangeThe domain and range of the function f= ((1/1x2)) x ∈ R, x ≠ ± 1 are respectively (A) R 1, 1 (∞, 0) ∪ 1, ∞) (B) R, (∞, 0) ∪ 1



Answer In Algebra For Dani Wagas



Domain And Range Examples Domain And Range Of Functions
Create your account We are given the function f(x) = 2x2−3x−1 f ( x) = 2 x 2 − 3 x − 1 We want to find theDomain and range graph The graph of f(x)=x2 f ( x) = x 2 (red) has the same domain (input values) as the graph of f(x)=− 1 12x3 f ( x) = − 1 12 x 3 (blue) since all real numbers can be input values However, the range of the red graph is restricted to only f(x)≥0 f ( x) ≥ 0, or y y values above or equal to 0 0 2x 1 = 0 2x = 1 x = 1 / 2 Therefore , Domain = R { 1/2 } All real numbers are used as range here For getting solve the denominator of the given fraction and reduce the value of x from the real number "R" Hope it helps you dude



2 A Find The Domain And Range Of Each Function Chegg Com




Find Domain And Range Of Function Let A 2 1 0 1 2 And F A Gtz Be Given By F X X 2 2x Youtube
Range The set of all the outputs of a function is known as the range of the function or after substituting the domain, the entire set of all values possible as outcomes of the dependent variable For eg the range of the function F is {19, 1987, 1992, 1996} On the other hand, the whole set B is known as the codomain of the functionRange The out comes or values that we get for y is known as range Domain for given function f (x) = x 3 For any real values of x, f (x) will give defined values Hence the domain is R Since we have absolute sign, we must get only positive values by applying any positive and negative values for x in the given function Example 15 Define the real valued function f R – {0} → R defined by f (x) = 1/𝑥 x ∈ R – {0} Complete the Table given below using this definition What is the domain and range of this function?




Find The Domain And The Range Of The Real Function F X 1 X 3



Graphing Cube Root Functions
The domain is all real numbers less than or equal to 4, and the range is all real numbers such that –3 ≤ x ≤ 1Given f(x) = 1/𝑥 , x ∈ R – {0} Finding f(x) at different values of x f(−2) = 1/(−2) 1 Confirm that you have a quadratic function A quadratic function has the form ax 2 bx c f (x) = 2x 2 3x 4 The shape of a quadratic function on a graph is parabola pointing up or down There are different methods to calculating the range of a function depending on the type you are working with
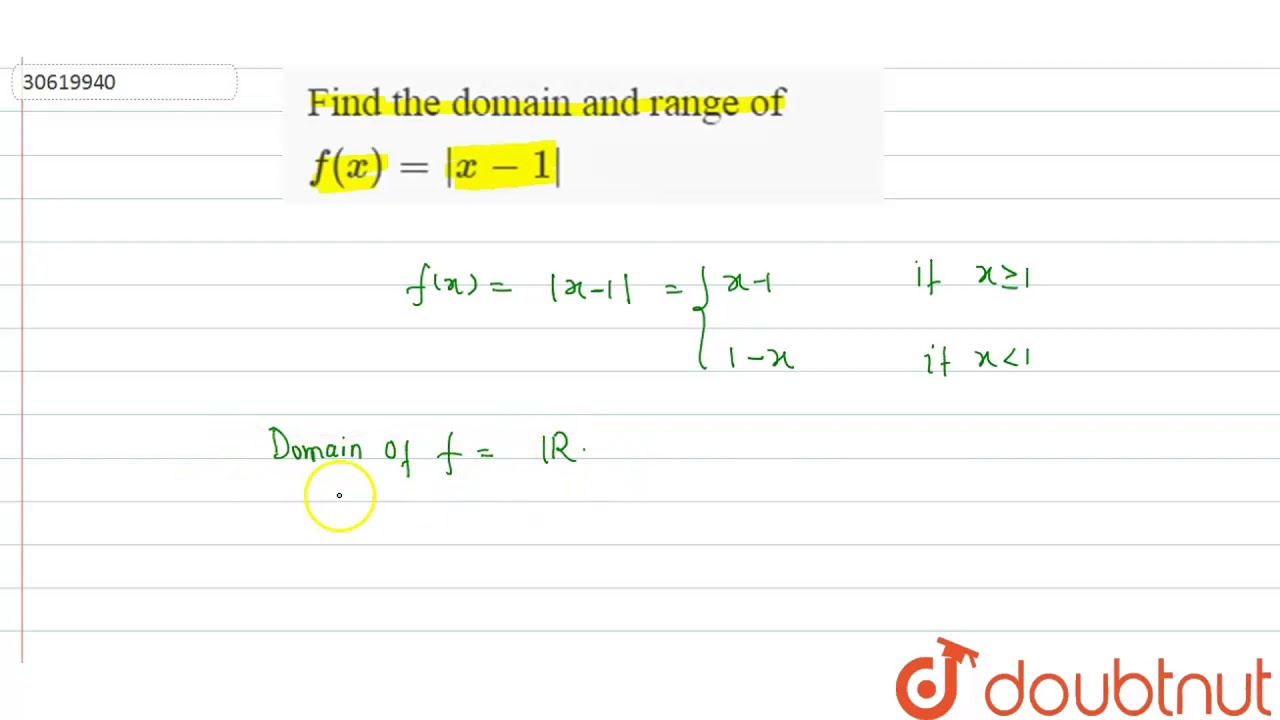



Find The Domain And Range Of F X X 1 Youtube
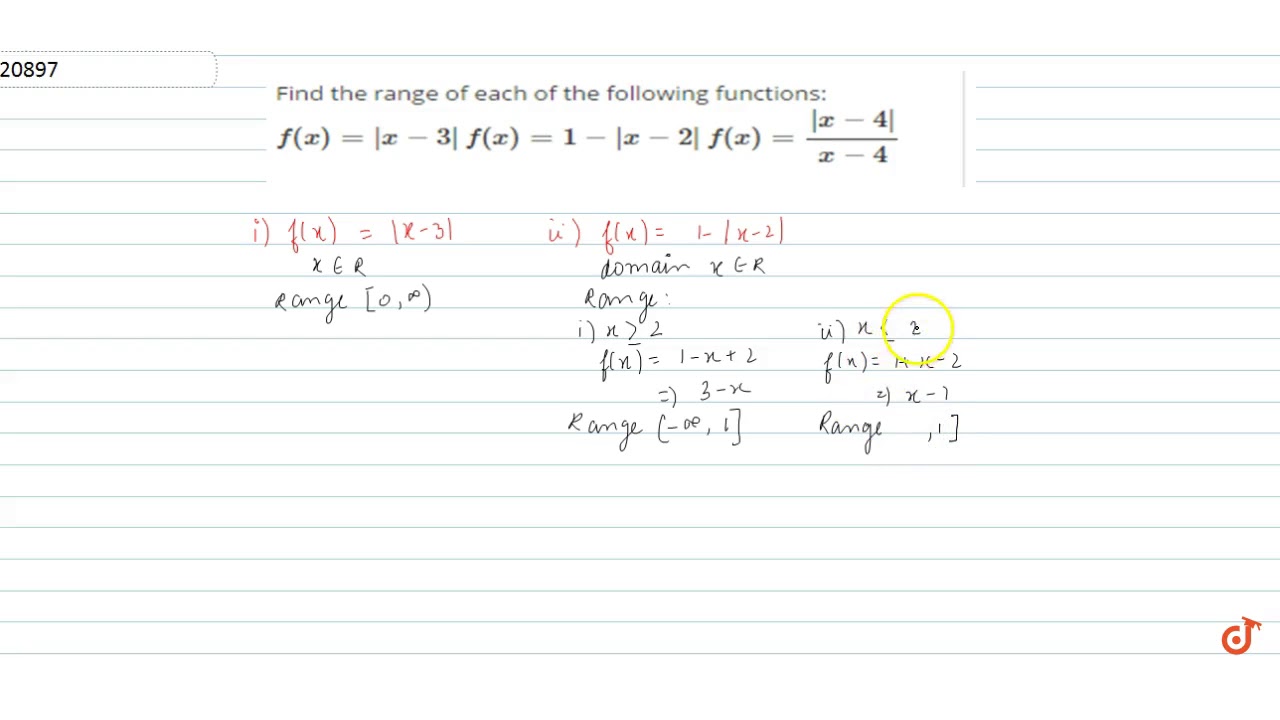



Find The Range Of Each Of The Following Functions F X X 3 F X 1 X 2 F X X 4 Youtube
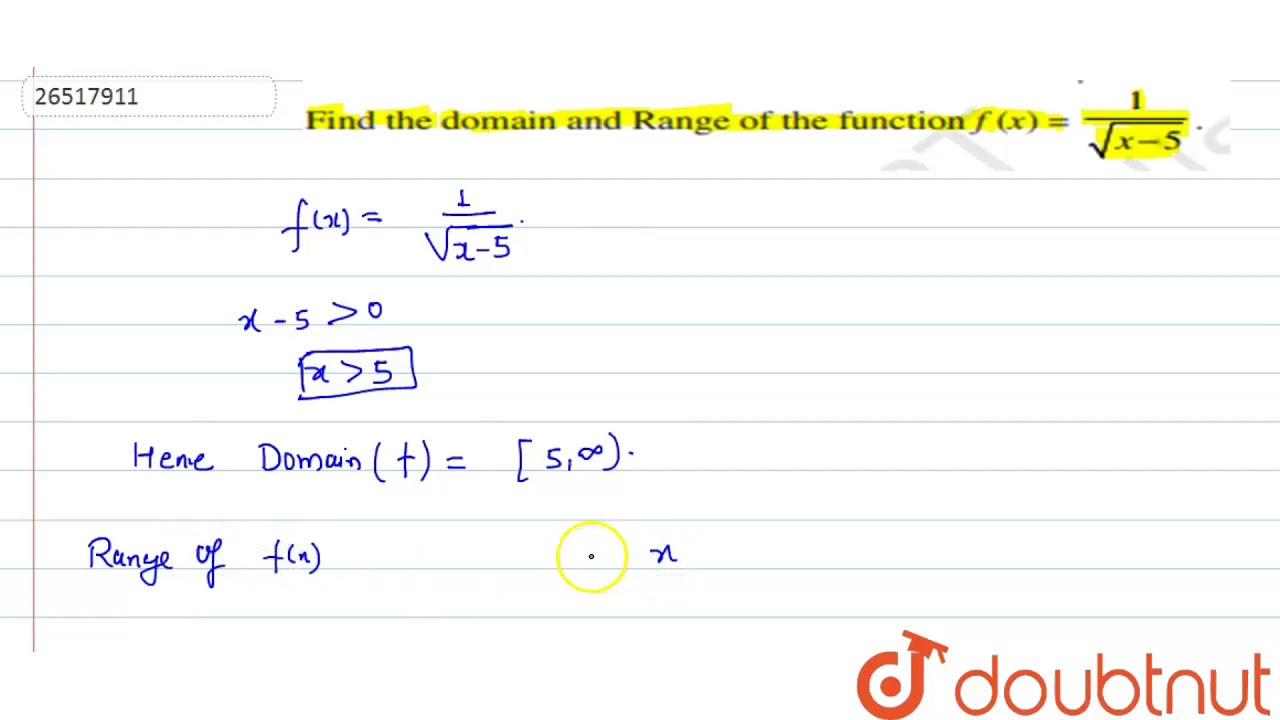
Given f (x) = 1/√x−5 To find the domain and range of function Explanation So, the domain of a function consists of all the first elements of all the ordered pairs, ie, x, so we have to find the values of x to get the required domain Given, f (x) = 1/√x−5 Now for real value of x5≠0 and x5>0 ⇒ x≠5 and x>5 Hence the domain of f = (5, ∞)There are no restrictions on the inputRational functions f(x) = 1/x have a domain of x ≠ 0 and a range of x ≠ 0 If you have a more complicated form, like f(x) = 1 / (x – 5), you can find the domain and range with the inverse function or a graph See Rational functions Sine functions and cosine functions have a domain of all real numbers and a range of 1 ≤ y ≤ 1
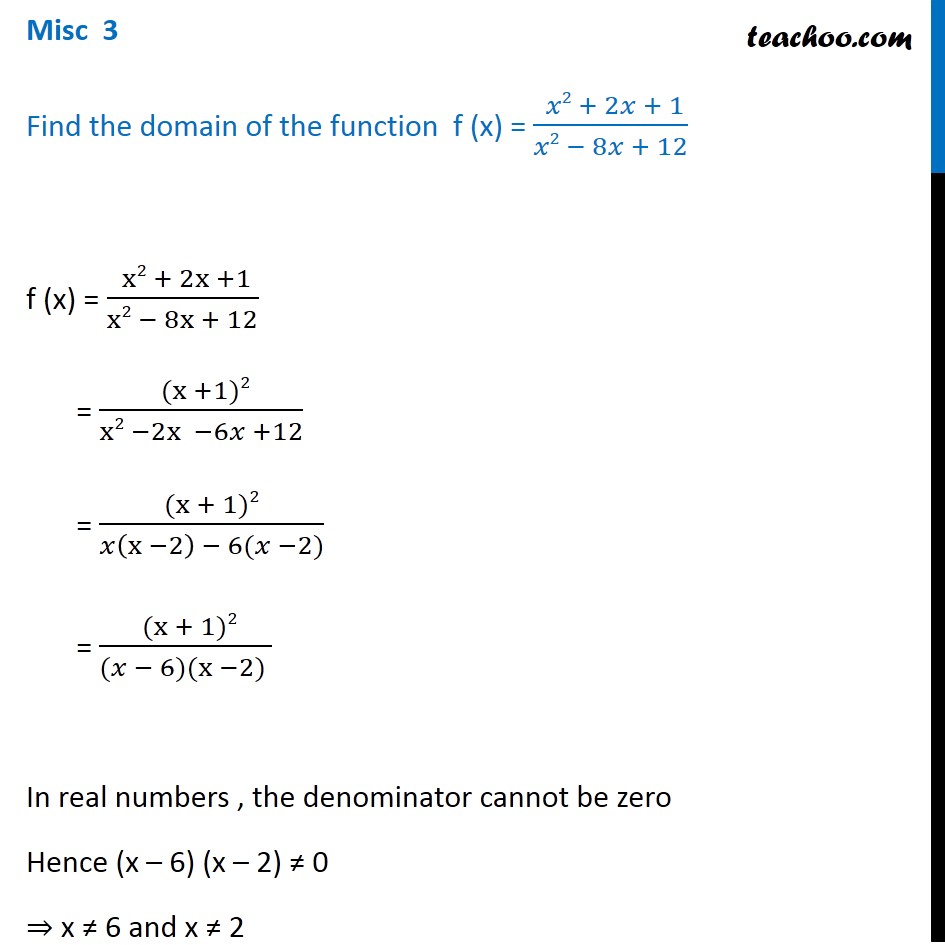



Misc 3 Find Domain Of F X X2 2x 1 X2 8x 12




Introduction To Function Domain And Range Mohd Noor
Solution For Find the domain and range of the following functions (i) f(x)=sqrt(2x3) " (ii) " f(x) =(1)/(x2) (iii) f(x) =x^(2) 3 " Misc 4 Find the domain and the range of the real function f defined by f(x) = √((𝑥−1)) It is given that the function is a real function Hence, both its domain and range should be real numbers x can be a number greater 1 Here, f(x) is always positive, Minimum value of f(x) is 0,What is the domain and range of the following rational expression?
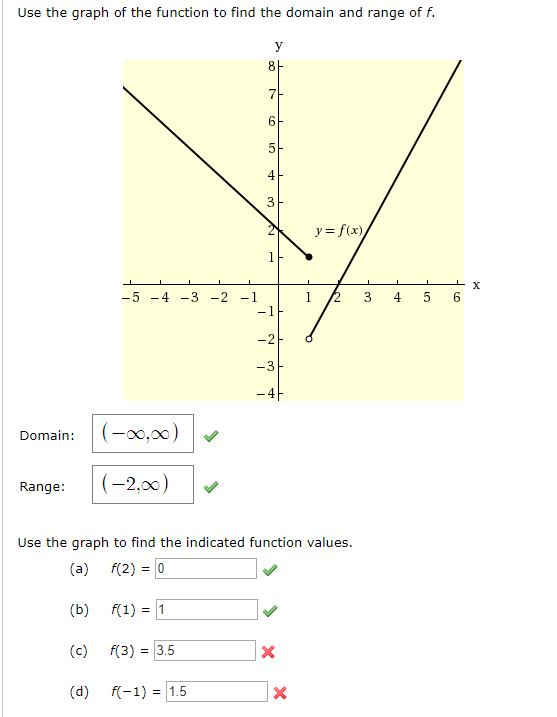



Use The Graph Of The Function To Find The Domain And Chegg Com
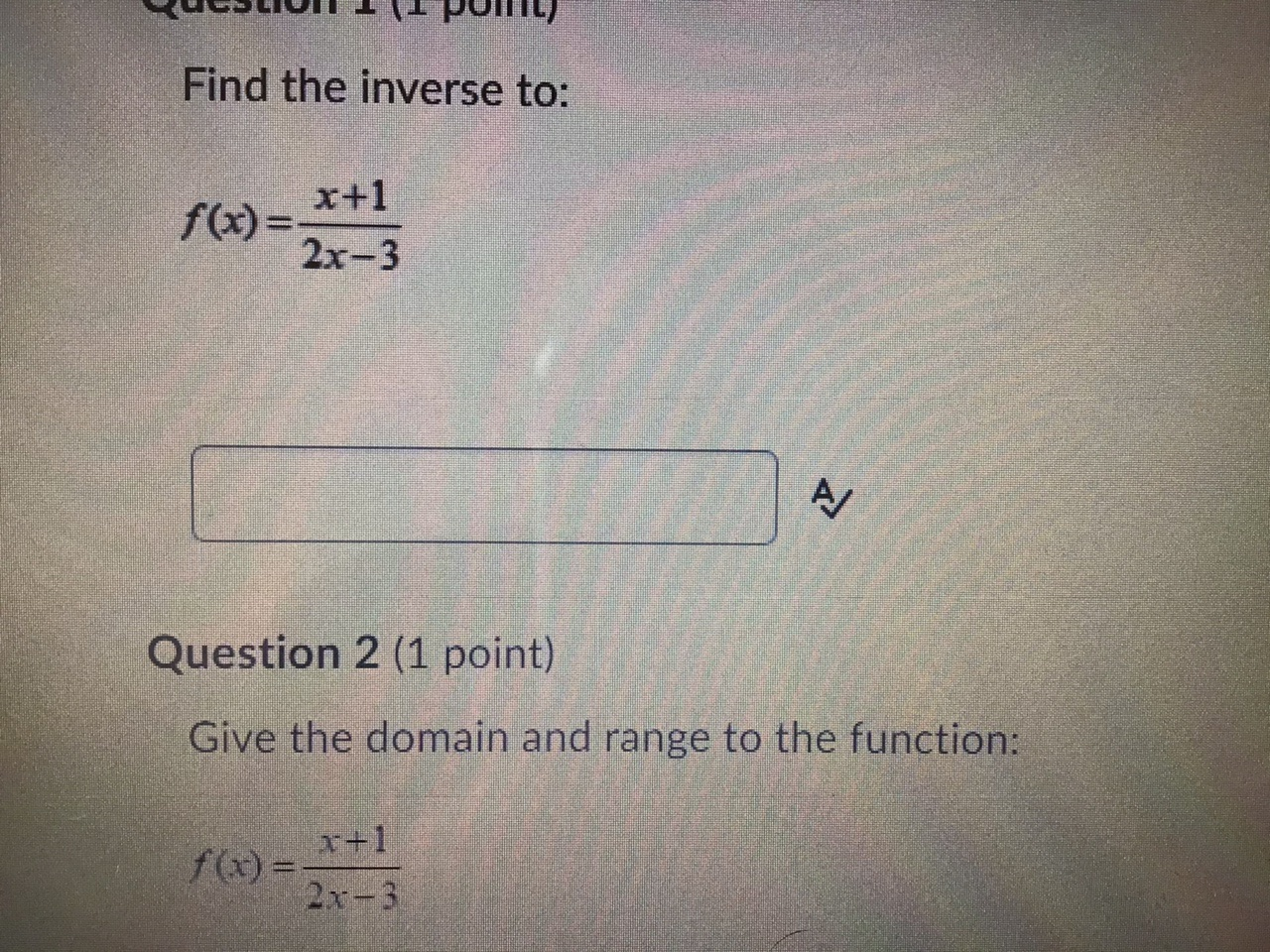



Find The Inverse To F X X 1 2x 3 Question 2 1 Chegg Com
To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW Find domain and range of `f(x)=x/(1x^2)`What are the domain and range of the realvalued function f(x) = −3x 2 6x 1?Find the domain and range of the function f (x) = 2 − sin3x1 Solution Solution f (x) = 2 − sin3x1 Here, 2 − sin3x can never be zero as sin3x will always less than 2 ∴ Domain of f (x) will be x ∈ R Now, f (x) will be maximum when 2 − sin3x is minimum 2 − sin3x will be minimum when sin3x = 1



Q Tbn And9gcqgqrwpnxjwt0kskruy6guwbb9vrtxcyvozrczh7yys7zu6fw5a Usqp Cau



Determine Domain And Range From A Graph College Algebra
Find the domain of 1/(e^(1/x)1) function domain square root of cos(x) Calc Find the functions a) f o g, b) g o f, c) f o f, d) g o g, and their domains f(x)= square root of x, g(x)= cube root of 1x These are my answers, but I am not sure about them and I only figured out one domain that is the partFree functions domain calculator find functions domain stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy




3 6 Warm Up Find The Initial Point State The Domain Range And Compare To The Parent Function F X X Y 3 X 1 Y 1 2 X Y X 1 Ppt Video Online Download



Graphing The Basic Functions




How To Find Domain And Range From A Graph Video Khan Academy



Determine Domain And Range From A Graph College Algebra



Boitz Weebly Com Uploads 2 5 6 5 Key 2 1 Pdf
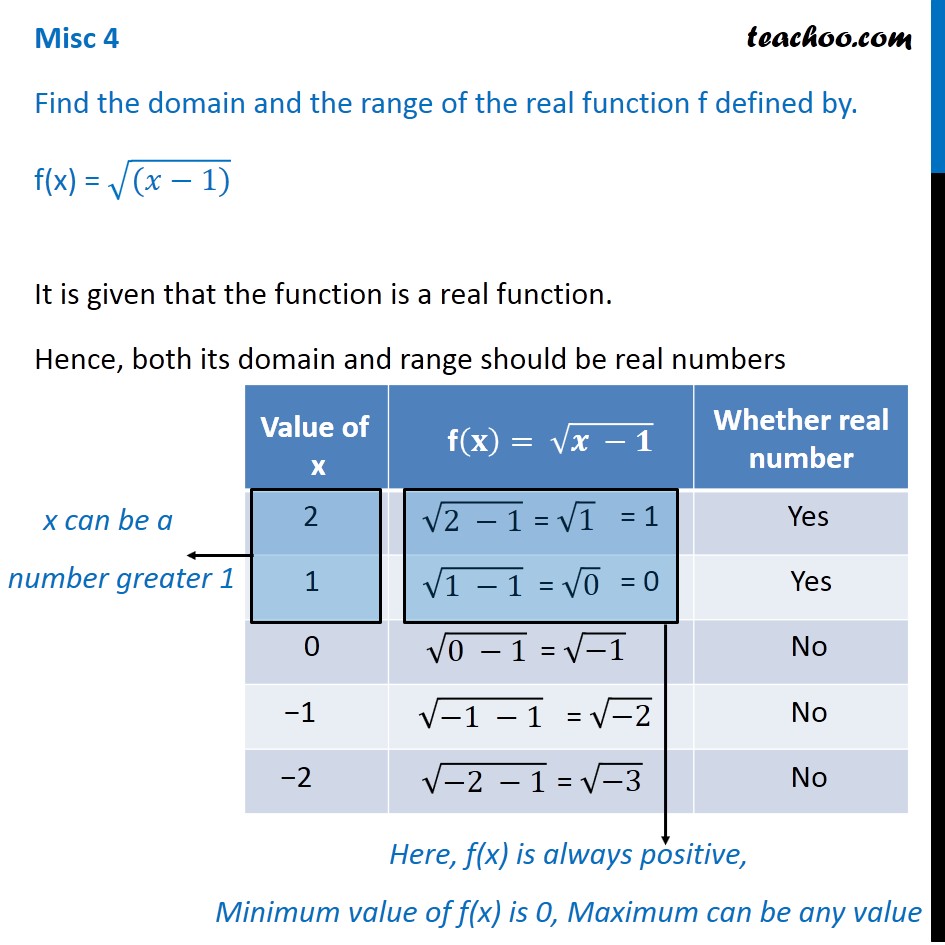



Misc 4 Find Domain And Range Of F X Root X 1 Chapter 2



Find Inverse And Its Domain And Range F X X Chegg Com



View Question Domain And Range Questions Help
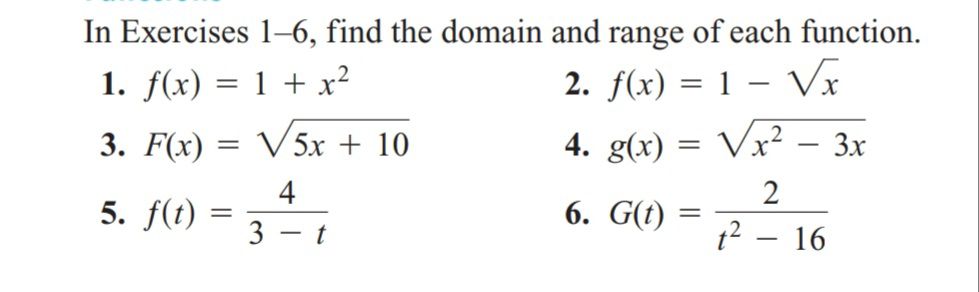



Answered In Exercises 1 6 Find The Domain And Bartleby



For The Function F X 3 X 1 2 2 Identify The Vertex Domain And Range The Vertex Is 1 2 Brainly Com
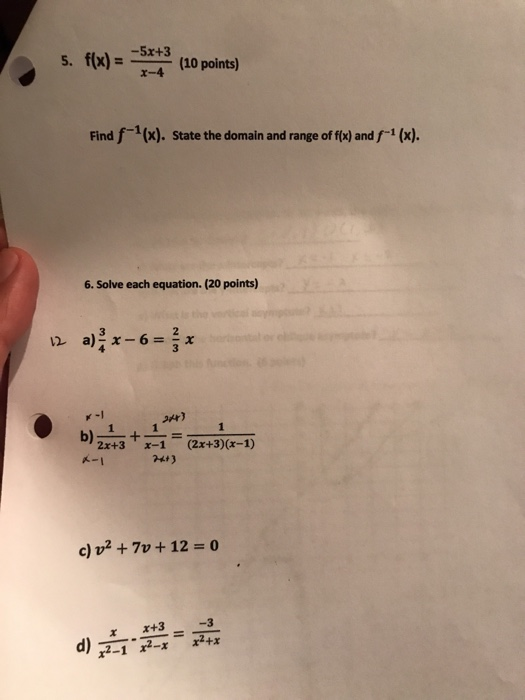



5 Fx F X 5x 3 10 Points X 4 Findf 1 X State Chegg Com
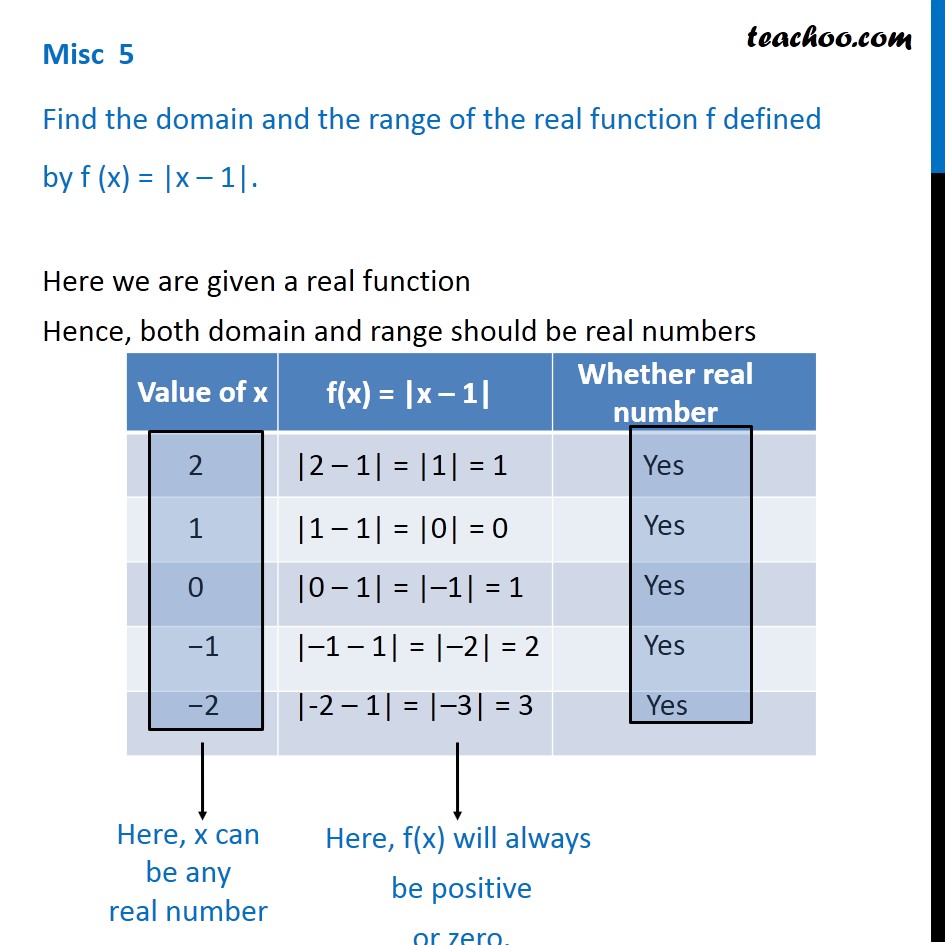



Misc 5 Find Domain And Range Of F X X 1 Class 11




How Do You Find The Domain And Range Of Y 3x 2 Socratic




Find The Domain And Range Of The Following Functions I F X X 2 Ii F X X 1 3 X




Domain And Range Of F X X 3 X 3 Are Respectively



U2l1 Domain And Range
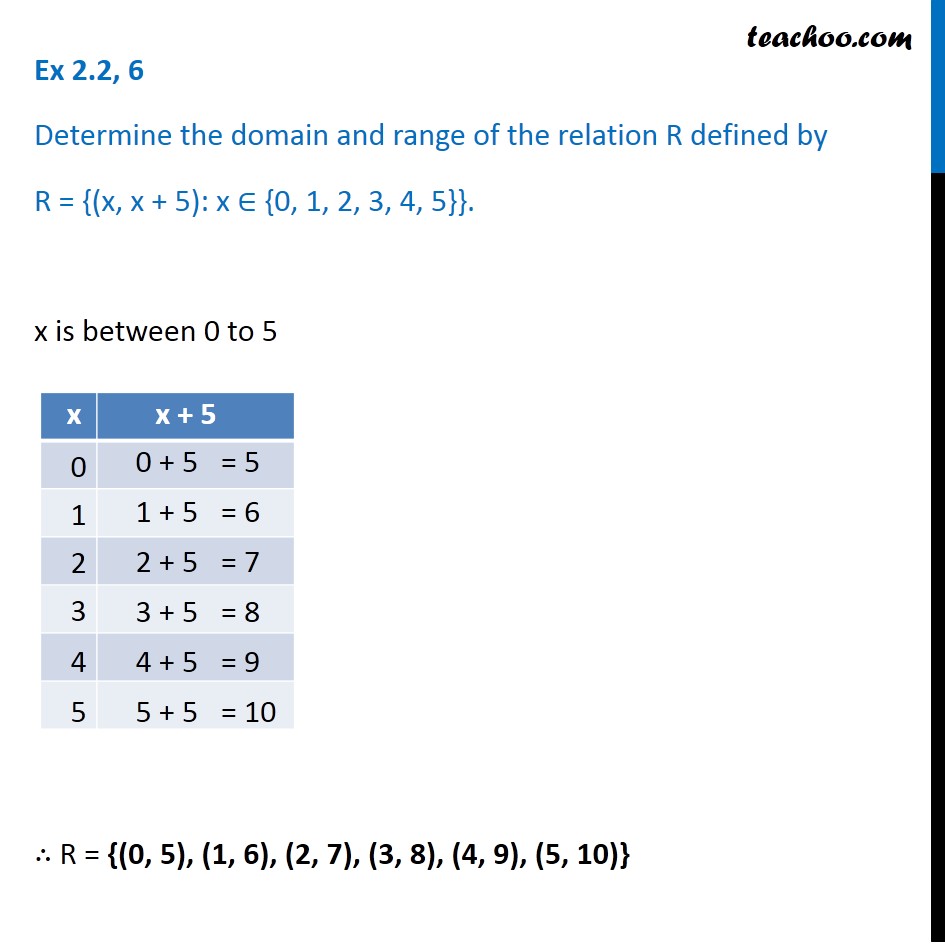



Ex 2 2 6 Determine Domain And Range Of R X X 5
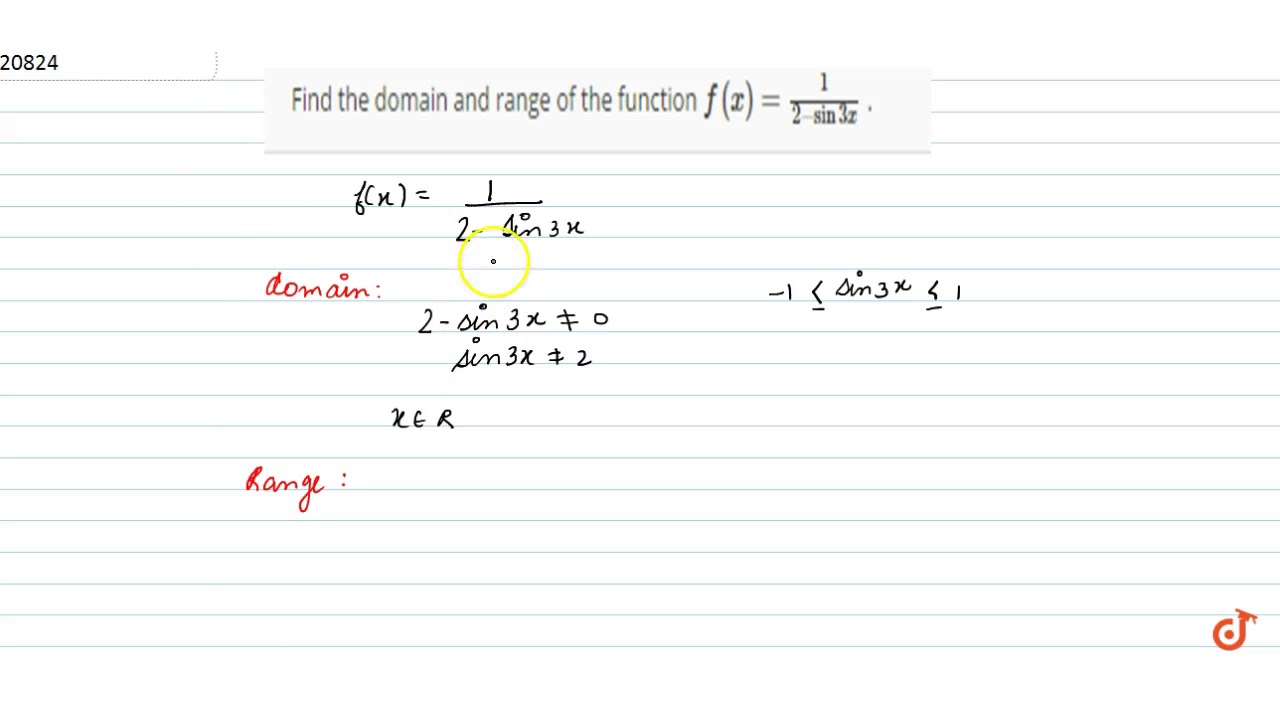



Find The Domain And Range Of The Function F X 1 2 Sin3x Youtube
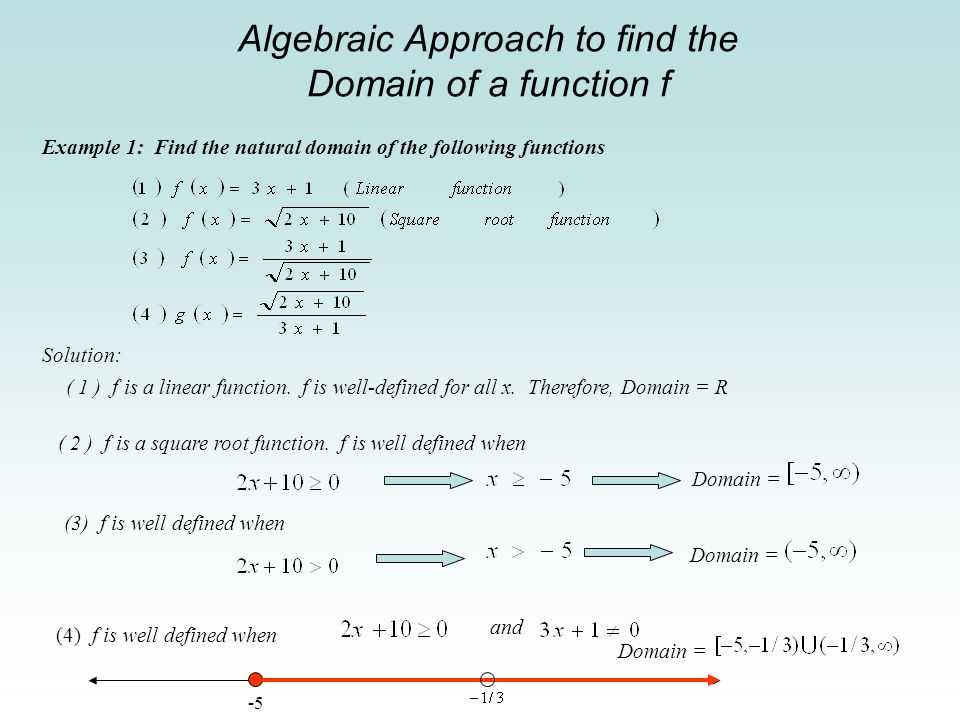



Interpretation Of Domain And Range Of A Function F Ppt Download




6 Ways To Find The Domain Of A Function Wikihow




Given That F X 1 2x 3 Find F 1 The Domain Of Chegg Com



Domain And Range Of Rational Functions




Tutorial Function Domain And Range Pdf Variable Mathematics Domain Of A Function




Find The Domain And Range Of Each Of The Following Functions F X X 2 X 1 X 2 4x 3



Search Q How To Find The Domain Of A Function Tbm Isch




Exercise 1 3 Functions Problem Questions With Answer Solution




How Do You Graph F X 2 X 1 3 And State The Domain And Range Socratic



What Is The Domain And Range Of 1 X 3 Quora




Find The Domain Or Range Of Following Function Tex F X Sqrt X 1 3 X Tex Brainly In



Advanced Algebra And Trig



Find The Domain And Range Of The Function F X 1 Sqrt X 5 Youtube



Domain And Range Of Exponential And Logarithmic Functions



Q1 Find The Natural Domain And Range And Also Sketch Chegg Com



What Is The Domain And Range For The Following Function And Its Inverse F X 3x 1 2 Mathskey Com




Finding Domain And Range




2 Find Then Espai 1 Y Log 5 V2 Sinx Cos X 3 Find The




Domain And Range Of Functions



Draw The Graph Of The Function F R R Defined By F X X 3 Find Its Domain And Range Sarthaks Econnect Largest Online Education Community




Domain And Range Of Functions
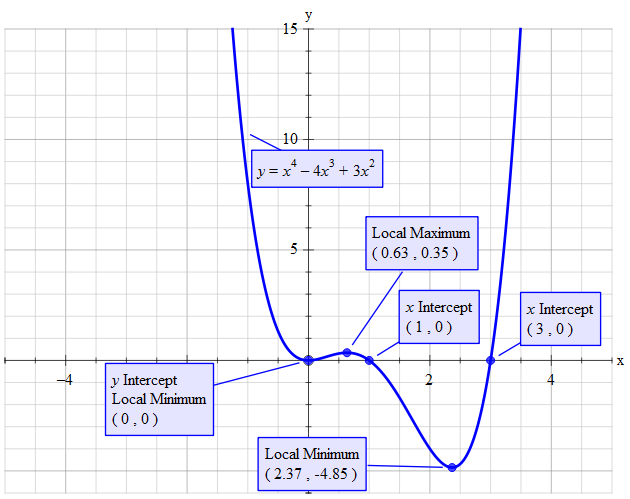



How Do You Find The Domain And Range Of F X X 4 4x 3 3x 2 Socratic
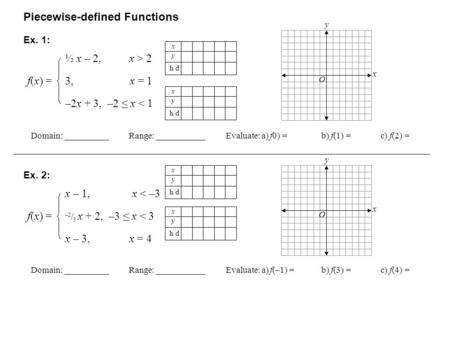



Section 3 2 Domain Range Ppt Download
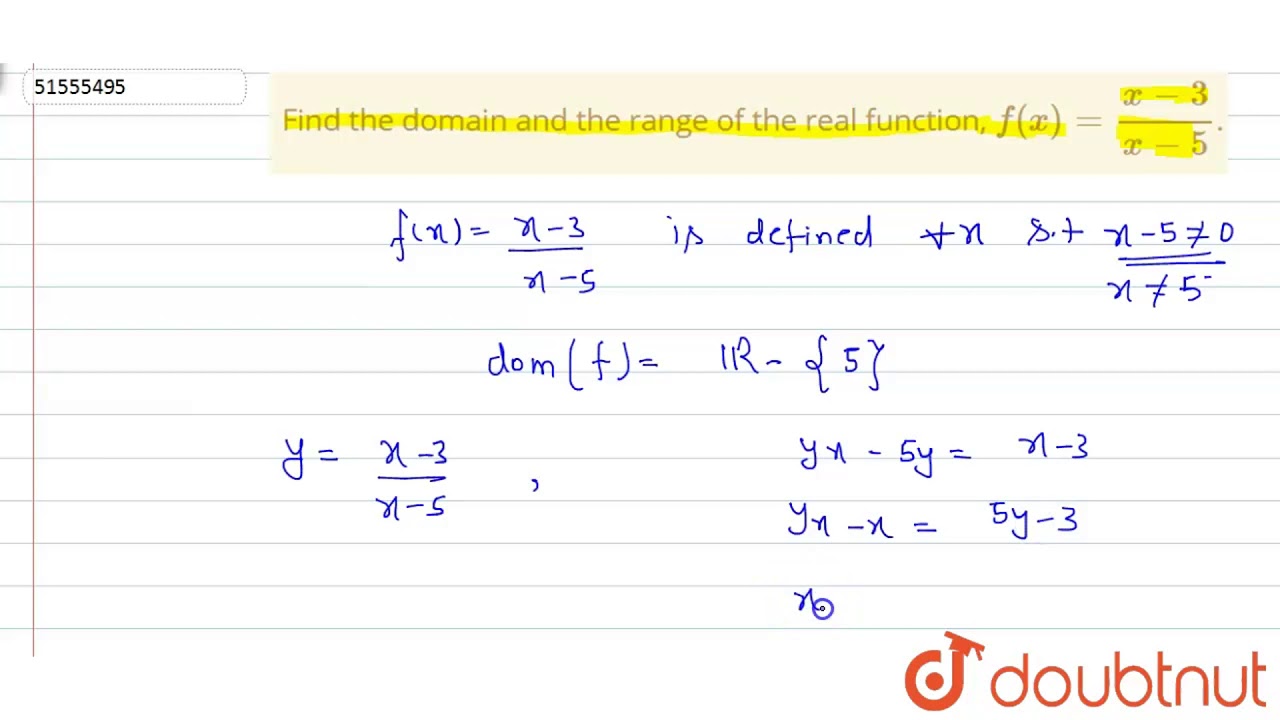



Find The Domain And The Range Of The Real Function F X X 3 X 5 Youtube




Find Domain And Range Of Real Functions 1 F X X 2 3 X 2 F X 1 Sqrt X 5 3 F X X 1 X 2
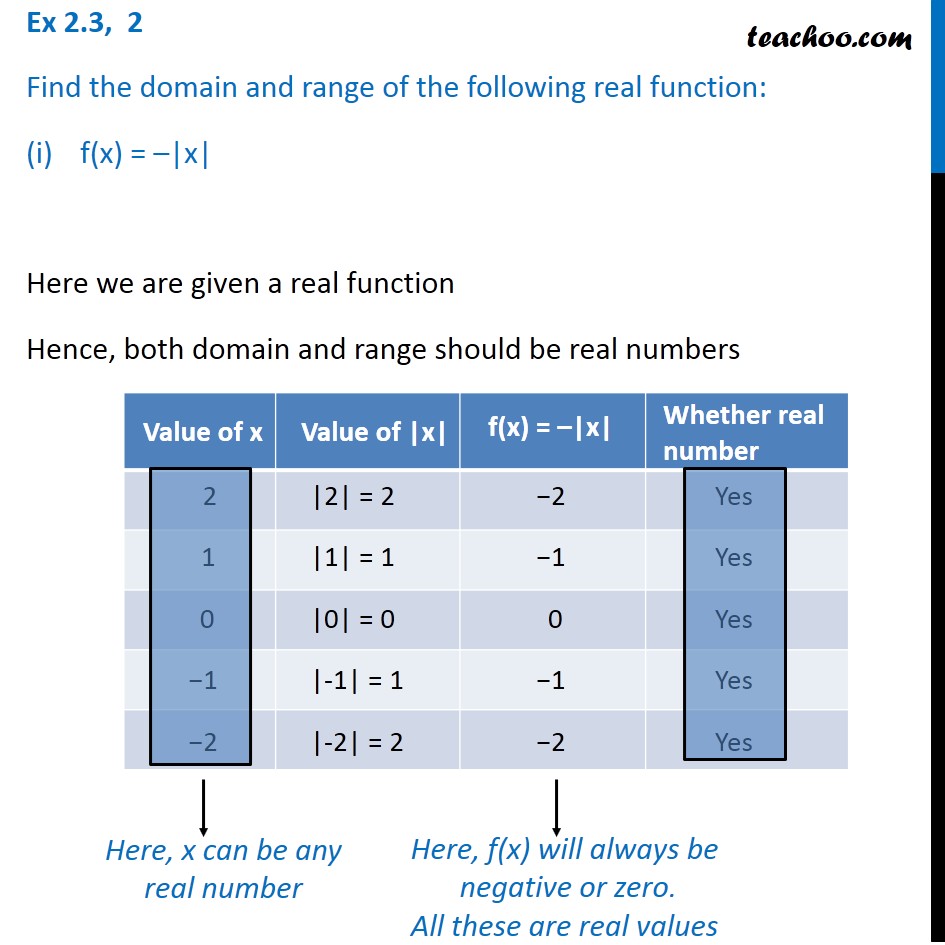



Ex 2 3 2 Find The Domain And Range Of The Function F X X




Determine Domain And Range From A Graph College Algebra




I Will Give Brainiestthe Graph Of The Function F X X 3 X 1 Is Shown Below What Is True Brainly Com



Solution Suppose That F X Log3 X 1 A State The Domain And Range Of F B Find A Formula For F 1 C Sketch The Graph Of F And F 1 Label All Important Points And Asymptotes




6 Ways To Find The Domain Of A Function Wikihow



Domain And Range Of Quadratic Functions Video Khan Academy




How To Find The Domain And Range Of A Function 14 Steps



Http Fsw01 c Cuny Edu Luis Fernandez01 Web Teaching Classes Math30 Nikos 30sp10 Funex Pdf



What Is The Domain And Range Of The Sequence F X X 1 X 3 Quora




Domain And Range Calculator Wolfram Alpha



Domain And Range Examples Domain And Range Of Functions



Www Math Colostate Edu Clayton Teaching M113f09 Homework Hw1solutions Pdf
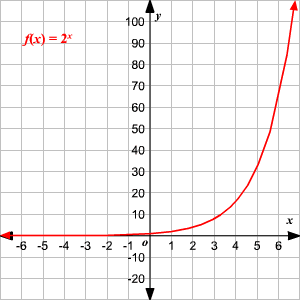



Domain And Range Of Exponential And Logarithmic Functions




Find The Domain And Range Of The Function F X 16 X 2 Donimain




Find Domain And Range Of Real Functions 1 F X X 2 3 X
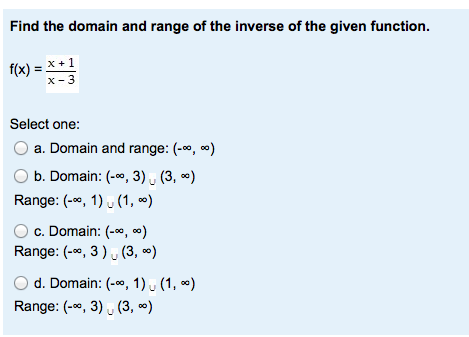



Find The Domain And Range Of The Inverse Of The Given Chegg Com
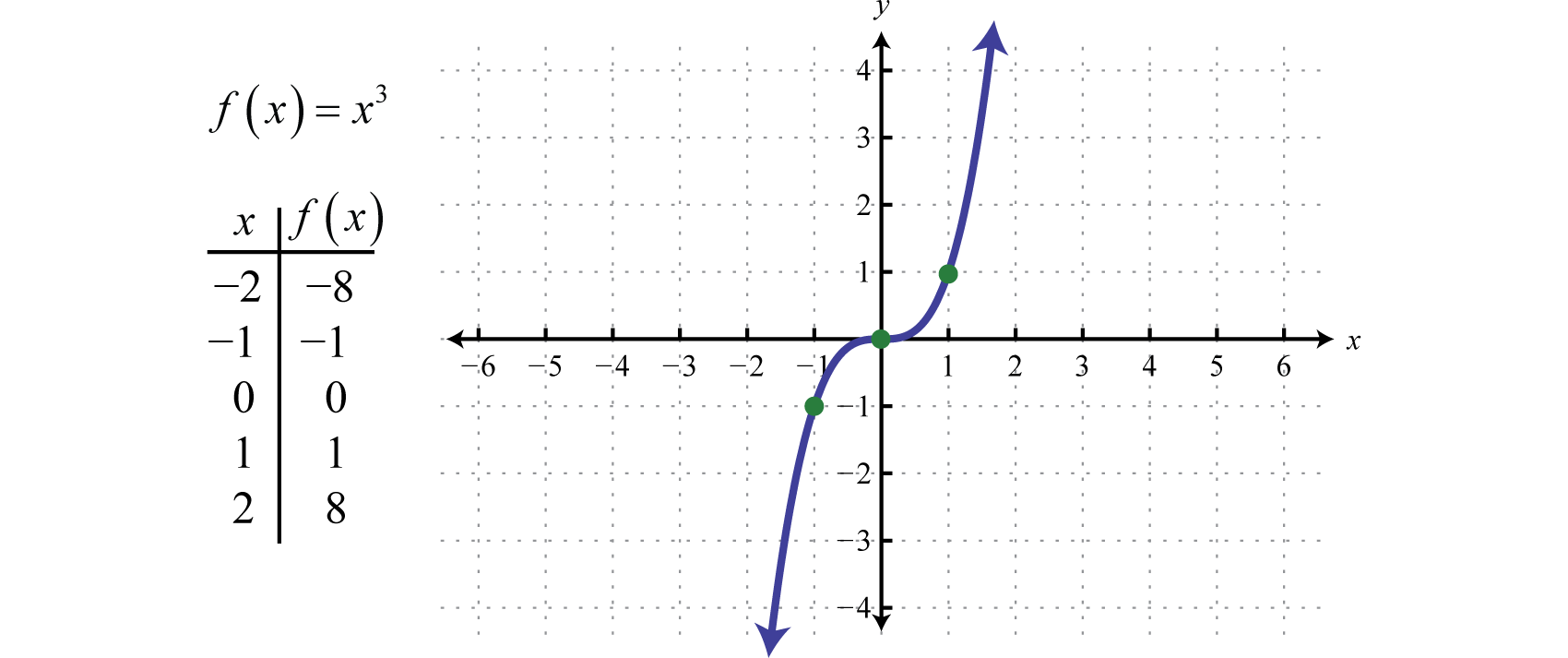



Graphing The Basic Functions




4 The Domain And Range Of F X Are 0 2 And Chegg Com




Find Domain And Range Of Real Functions 1 F X X 2 3 X 2 F X 1 Sqrt X 5 Youtube
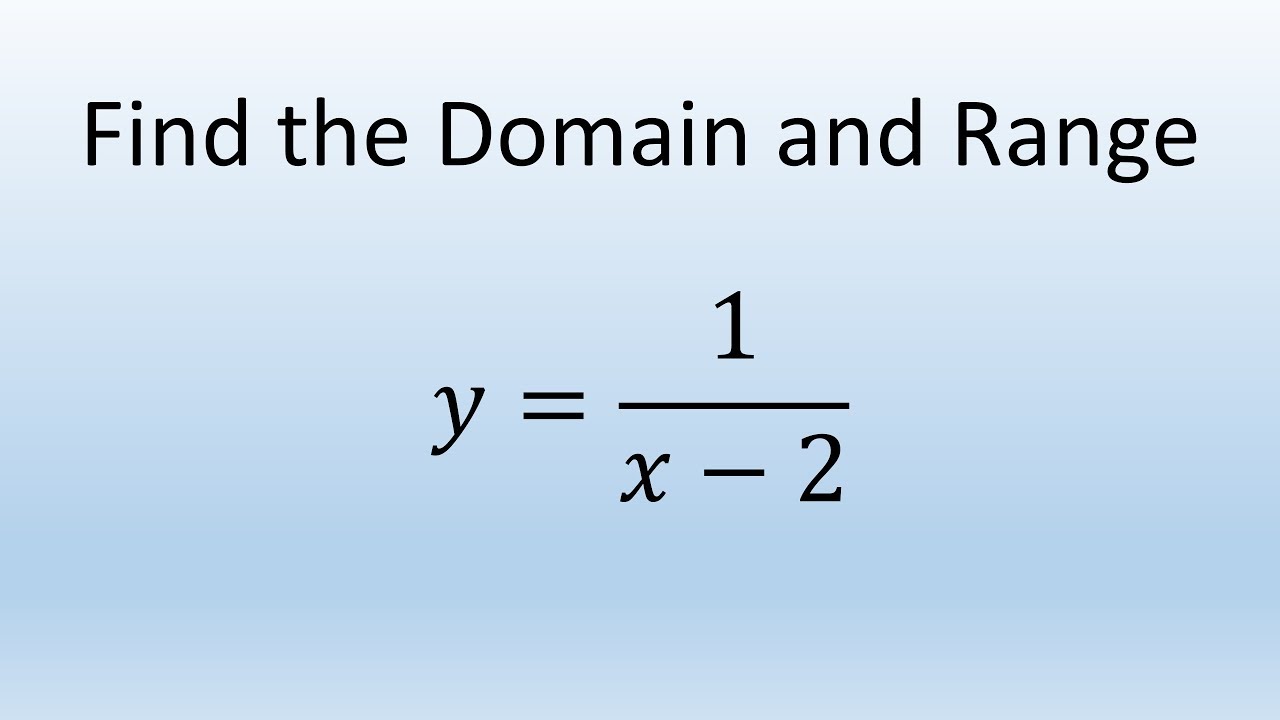



Find Domain And Range Of Function Y 1 X 2 Youtube
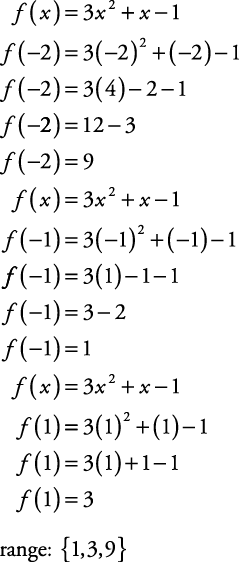



Functions




F X Log 1 3 H A Domain 0 Range B Domain 0 Homeworklib



How Do You Find The Domain And Range Of Y Sqrt 3x 5 Socratic
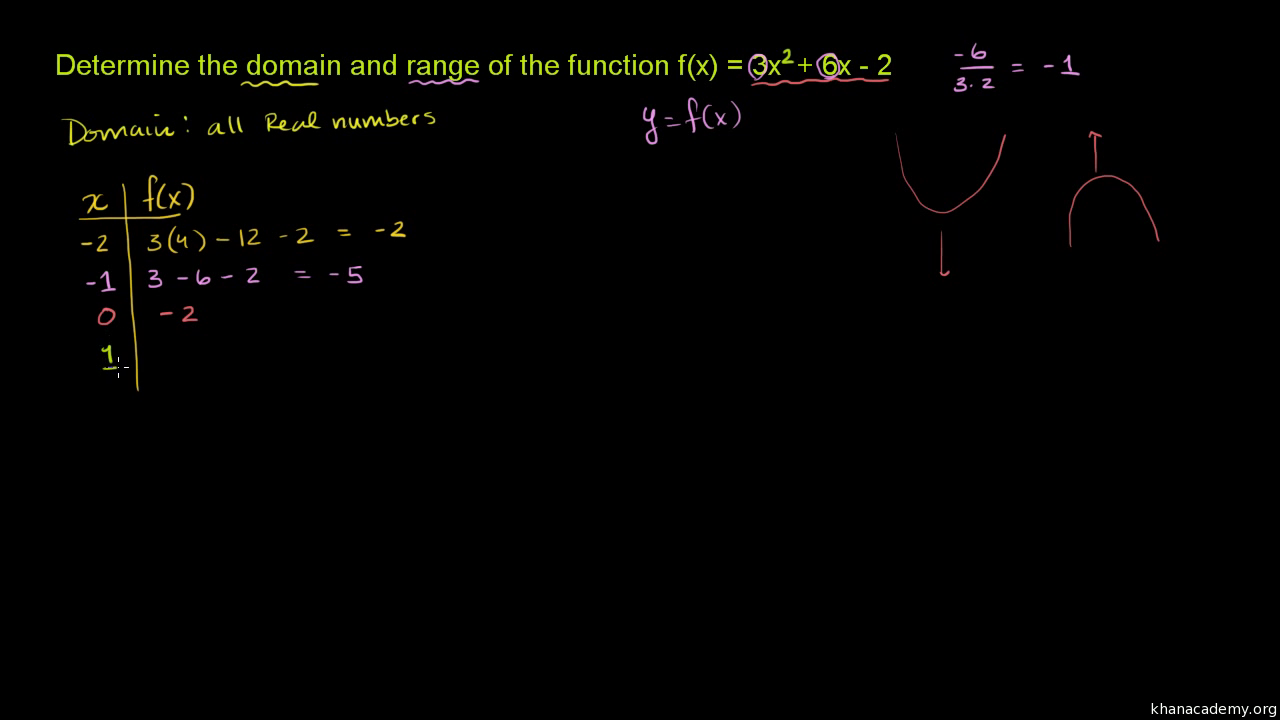



Domain And Range Of Quadratic Functions Video Khan Academy
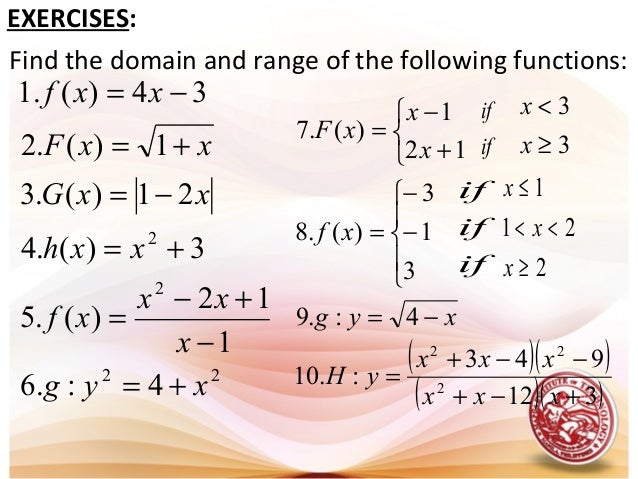



L1 Functions Domain Amp Range



3



Can You Sketch The Graph Of F X 2x 2 X 3 2x 3 And Give Its Domain Intercepts Asymptotes And Range Quora




Find The Domain And The Range Of The Real Function F X X 3 X 3




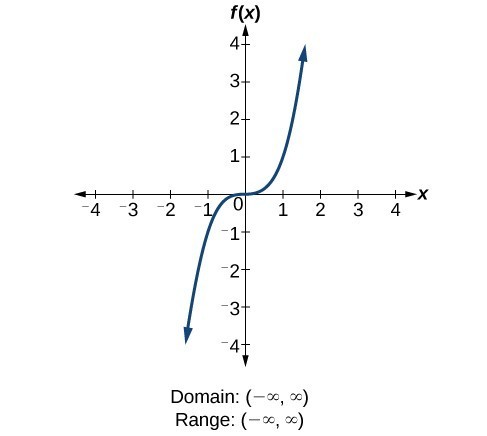
Find Domains And Ranges Of The Toolkit Functions Math 1314 College Algebra




Find Domain And Range F X X 3x 13 4 Maths Relations And Functions Meritnation Com




How To Find The Domain And Range Of A Function 14 Steps




Finding Domain And Range



What Is The Domain And Range Of F X X 1 X 2 1 X 3 Quora




What Is True About The Domain And Range Of The Function The Graph Of The Function F X X 2 X Brainly Com



1



0 件のコメント:
コメントを投稿